
On Wolfram|Alpha Quadratic Equation Cite this as:įrom MathWorld-A Wolfram Web Resource. Example: 3x2-2x-10 (After you click the example, change the Method to 'Solve By Completing the Square'.) Take the Square Root. Washington, DC: Hemisphere, pp. 123-131, 1987. There are different methods you can use to solve quadratic equations, depending on your particular problem. "The Quadratic Function and ItsĪtlas of Functions. Cambridge, England:Ĭambridge University Press, pp. 178-180, 1992. Recipes in FORTRAN: The Art of Scientific Computing, 2nd ed. "Quadratic and Cubic Equations." §5.6 in Numerical What are \(5\) methods of solving a quadratic equation Ans: We can solve the quadratic equations by using different methods given below: 1. Oxford,Įngland: Oxford University Press, pp. 91-92, 1996. FAQs on Methods of Solving Quadratic Equations. Is Mathematics?: An Elementary Approach to Ideas and Methods, 2nd ed.
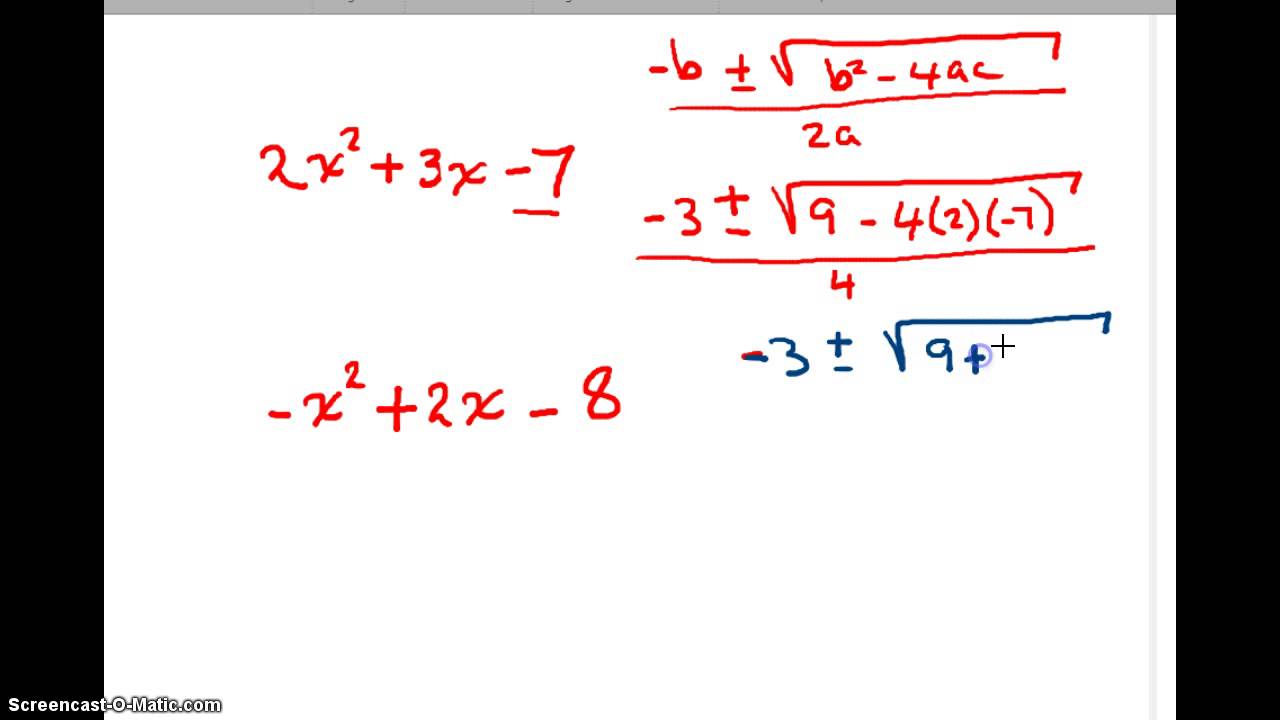
"Quadratic Equations."Īnd Polynomial Inequalities. Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables, 9th printing. Viète was among the first to replace geometric methods of solution with analytic ones, although he apparently did not grasp the idea of a general quadratic equation (Smith 1953, pp. 449-450).Īn alternate form of the quadratic equation is given by dividing (◇) through by : The Persian mathematiciansĪl-Khwārizmī (ca. 1025) gave the positive root of the quadratic formula, as statedīy Bhāskara (ca. 850) had substantially the modern rule for the positive root of a quadratic. The solution (for real numbers) is where the parabola cross the x-axis. Graphically, since a quadratic equation represents a parabola. Of them (Smith 1951, p. 159 Smith 1953, pp. 444-445). The solution of a quadratic equation is the value of x when you set the equation equal to zero. Depending on the values that b and c have, we will be talking about complete. Of the quadratic equations with both solutions (Smith 1951, p. 159 Smithġ953, p. 444), while Brahmagupta (ca. The quadratic equation is of the form ax2+bx+c0 where a is nonzero.
#Solve quadratic equation series#
(475 or 476-550) gave a rule for the sum of a geometric series that shows knowledge
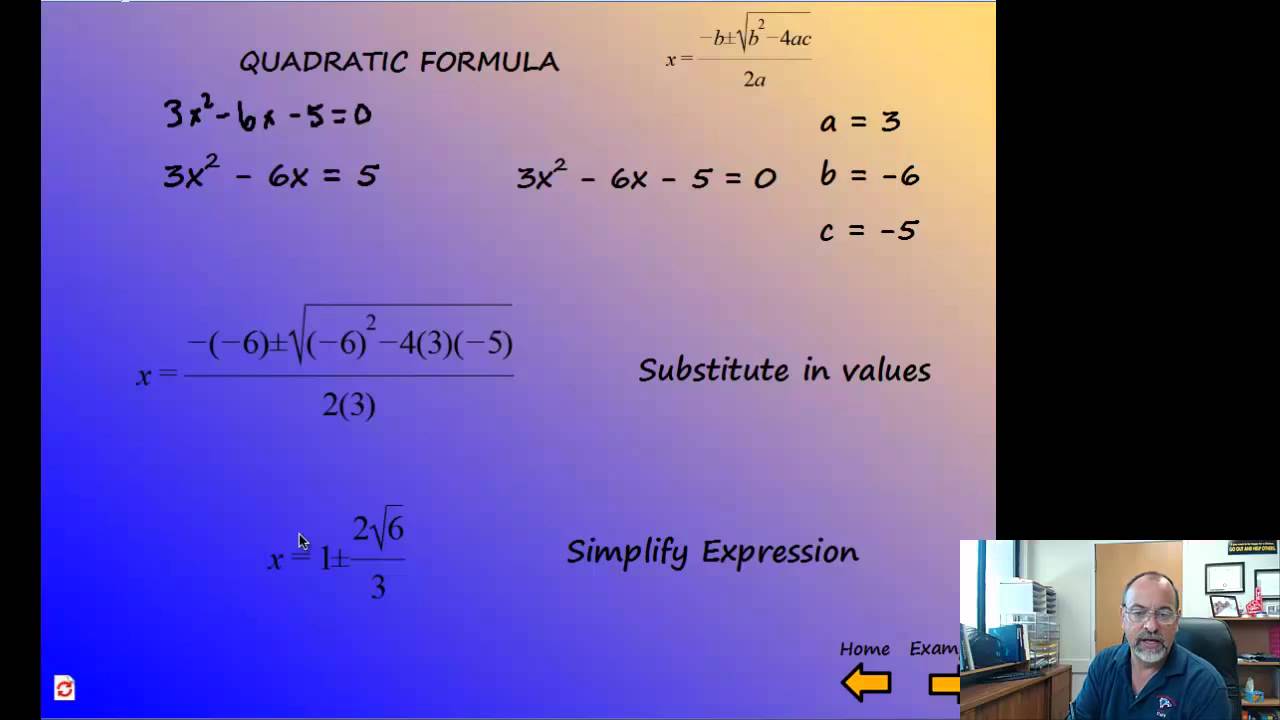
The method of solution (Smith 1953, p. 444). Solutions of the equation, but even should this be the case, there is no record of It is possible that certain altar constructions dating from ca. B This is the coefficient of the single powered term in the. (-B-SQRT(B2-4AC))/(2A) A This is the coefficient of the squared term in the quadratic equation. (-B+SQRT(B2-4AC))/(2A) This is the other possible solution. 210-290) solved the quadratic equation, but giving only one root, even whenīoth roots were positive (Smith 1951, p. 134).Ī number of Indian mathematicians gave rules equivalent to the quadratic formula. There are two possible solutions for any quadratic equation. In his work Arithmetica, the Greek mathematician Diophantus The Greeks were able to solve the quadratic equation by geometric methods, and Euclid's (ca.


 0 kommentar(er)
0 kommentar(er)
